Abstract:

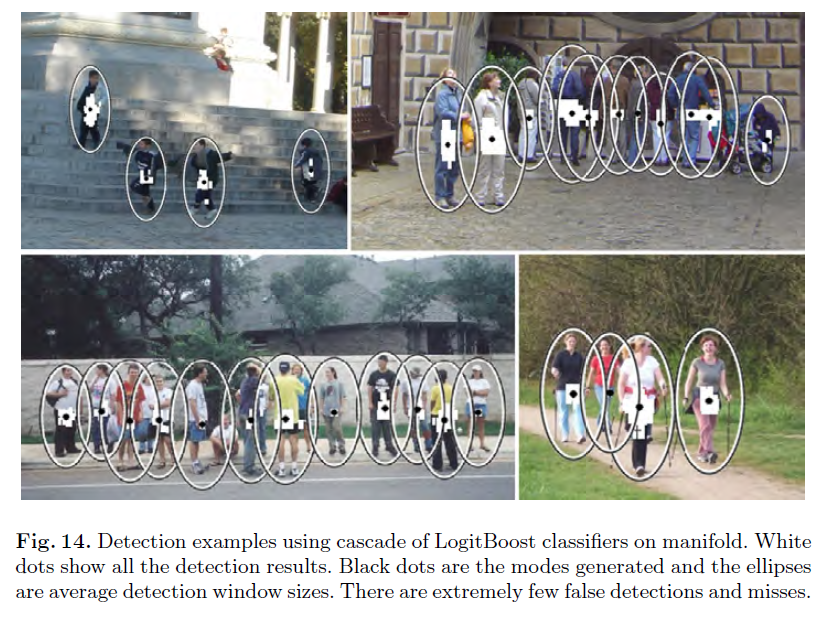
Mathematical formulation of certain natural phenomena exhibits group structure on topological spaces that resemble the Euclidean space only on a small enough scale, which prevents incorporation of conventional inference methods that require global vector norms. More specifically in computer vision, such underlying notions emerge in differentiable parameter spaces. Here, two Riemannian manifolds including the set of affine transformations and covariance matrices are elaborated and their favorable applications in distance computation, motion estimation, object detection and recognition problems are demonstrated after reviewing some of the fundamental preliminaries.